Outliers (24 page)
If you were a peasant farmer in Southern China, by contrast, you didn’t sleep through the winter. In the short break marked by the dry season, from November through February, you busied yourself with side tasks. You made bamboo baskets or hats and sold them in the market. You repaired the dikes in your rice paddy, and rebuilt your mud hut. You sent one of your sons to work in a nearby village for a relative. You made tofu and dried bean curd and caught snakes (they were a delicacy) and trapped insects. By the time
lahp cheun
(the “turning of the spring”) came, you were back in the fields at dawn. Working in a rice field is ten to twenty times more labor-intensive than working on an equivalent-size corn or wheat field. Some estimates put the annual workload of a wet-rice farmer in Asia at
three thousand
hours a year.
4.
Think, for a moment, about what the life of a rice farmer in the Pearl River Delta must have been like. Three thousand hours a year is a staggering amount of time to spend working, particularly if many of those hours involve being bent over in the hot sun, planting and weeding in a rice paddy.
What redeemed the life of a rice farmer, however, was the nature of that work. It was a lot like the garment work done by the Jewish immigrants to New York. It was
meaningful
. First of all, there is a clear relationship in rice farming between effort and reward. The harder you work a rice field, the more it yields. Second, it’s complex work. The rice farmer isn’t simply planting in the spring and harvesting in the fall. He or she effectively runs a small business, juggling a family workforce, hedging uncertainty through seed selection, building and managing a sophisticated irrigation system, and coordinating the complicated process of harvesting the first crop while simultaneously preparing the second crop.
And, most of all, it’s autonomous. The peasants of Europe worked essentially as low-paid slaves of an aristocratic landlord, with little control over their own destinies. But China and Japan never developed that kind of oppressive feudal system, because feudalism simply can’t work in a rice economy. Growing rice is too complicated and intricate for a system that requires farmers to be coerced and bullied into going out into the fields each morning. By the fourteenth and fifteenth centuries, landlords in central and Southern China had an almost completely hands-off relationship with their tenants: they would collect a fixed rent and let farmers go about their business.
“The thing about wet-rice farming is, not only do you need phenomenal amounts of labor, but it’s very exacting,” says the historian Kenneth Pomerantz. “You have to care. It really matters that the field is perfectly leveled before you flood it. Getting it close to level but not quite right makes a big difference in terms of your yield. It really matters that the water is in the fields for just the right amount of time. There’s a big difference between lining up the seedlings at exactly the right distance and doing it sloppily. It’s not like you put the corn in the ground in mid-March and as long as rain comes by the end of the month, you’re okay. You’re controlling all the inputs in a very direct way. And when you have something that requires that much care, the overlord has to have a system that gives the actual laborer some set of incentives, where if the harvest comes out well, the farmer gets a bigger share. That’s why you get fixed rents, where the landlord says, I get twenty bushels, regardless of the harvest, and if it’s really good, you get the extra. It’s a crop that doesn’t do very well with something like slavery or wage labor. It would just be too easy to leave the gate that controls the irrigation water open a few seconds too long and there goes your field.”
The historian David Arkush once compared Russian and Chinese peasant proverbs, and the differences are striking. “If God does not bring it, the earth will not give it” is a typical Russian proverb. That’s the kind of fatalism and pessimism typical of a repressive feudal system, where peasants have no reason to believe in the efficacy of their own work. On the other hand, Arkush writes, Chinese proverbs are striking in their belief that “hard work, shrewd planning and self-reliance or cooperation with a small group will in time bring recompense.”
Here are some of the things that penniless peasants would say to one another as they worked three thousand hours a year in the baking heat and humidity of Chinese rice paddies (which, by the way, are filled with leeches):
“No food without blood and sweat.”
“Farmers are busy; farmers are busy; if farmers weren’t busy, where would grain to get through the winter come from?”
“In winter, the lazy man freezes to death.”
“Don’t depend on heaven for food, but on your own two hands carrying the load.”
“Useless to ask about the crops, it all depends on hard work and fertilizer.”
“If a man works hard, the land will not be lazy.”
And, most telling of all: “No one who can rise before dawn three hundred sixty days a year fails to make his family rich.”
Rise before dawn?
360 days a year?
For the !Kung leisurely gathering mongongo nuts, or the French peasant sleeping away the winter, or anyone else living in something other than the world of rice cultivation, that proverb would be unthinkable.
This is not, of course, an unfamiliar observation about Asian culture. Go to any Western college campus and you’ll find that Asian students have a reputation for being in the library long after everyone else has left. Sometimes people of Asian background get offended when their culture is described this way, because they think that the stereotype is being used as a form of disparagement. But a belief in work ought to be a thing of beauty. Virtually every success story we’ve seen in this book so far involves someone or some group working harder than their peers. Bill Gates was addicted to his computer as a child. So was Bill Joy. The Beatles put in thousands of hours of practice in Hamburg. Joe Flom ground away for years, perfecting the art of takeovers, before he got his chance. Working really hard is what successful people do, and the genius of the culture formed in the rice paddies is that hard work gave those in the fields a way to find meaning in the midst of great uncertainty and poverty. That lesson has served Asians well in many endeavors but rarely so perfectly as in the case of mathematics.
5.
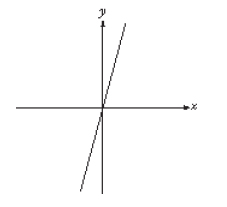
A few years ago, Alan Schoenfeld, a math professor at Berkeley, made a videotape of a woman named Renee as she was trying to solve a math problem. Renee was in her mid-twenties, with long black hair and round silver glasses. In the tape, she’s playing with a software program designed to teach algebra. On the screen are a
y
and an
x
axis. The program asks the user to punch in a set of coordinates and then draws the line from those coordinates on the screen. For example, when she typed in 5 on the
y
axis and 5 on the
x
axis, the computer did this:
At this point, I’m sure, some vague memory of your middle-school algebra is coming back to you. But rest assured, you don’t need to remember any of it to understand the significance of Renee’s example. In fact, as you listen to Renee talking in the next few paragraphs, focus not on what she’s saying but rather on how she’s talking and why she’s talking the way she is.
The point of the computer program, which Schoenfeld created, was to teach students about how to calculate the slope of a line. Slope, as I’m sure you remember (or, more accurately, as I’ll bet you don’t remember; I certainly didn’t), is rise over run. The slope of the line in our example is 1, since the rise is 5 and the run is 5.
So there is Renee. She’s sitting at the keyboard, and she’s trying to figure out what numbers to enter in order to get the computer to draw a line that is absolutely vertical, that is directly superimposed over the
y
axis. Now, those of you who remember your high school math will know that this is, in fact, impossible. A vertical line has an undefined slope. Its rise is infinite: any number on the
y
axis starting at zero and going on forever. It’s run on the
x
axis, meanwhile, is zero. Infinity divided by zero is not a number.
But Renee doesn’t realize that what she’s trying to do can’t be done. She is, rather, in the grip of what Schoenfeld calls a “glorious misconception,” and the reason Schoenfeld likes to show this particular tape is that it is a perfect demonstration of how this misconception came to be resolved.
Renee was a nurse. She wasn’t someone who had been particularly interested in mathematics in the past. But she had somehow gotten hold of the software and was hooked.
“Now, what I want to do is make a straight line with this formula, parallel to the
y
axis,” she begins. Schoenfeld is sitting next to her. She looks over at him anxiously. “It’s been five years since I did any of this.”
She starts to fiddle with the program, typing in different numbers.
“Now if I change the slope that way...minus 1... now what I mean to do is make the line go straight.”
As she types in numbers, the line on the screen changes.
“Oops. That’s not going to do it.”
She looks puzzled.
“What are you trying to do?” Schoenfeld asks.
“What I’m trying to do is make a straight line parallel to the
y
axis. What do I need to do here? I think what I need to do is change this a little bit.” She points at the place where the number for the
y
axis is. “That was something I discovered. That when you go from 1 to 2, there was a rather big change. But now if you get way up there you have to keep changing.”
This is Renee’s glorious misconception. She’s noticed the higher she makes the
y
axis coordinate, the steeper the line gets. So she thinks the key to making a vertical line is just making the
y
axis coordinate large enough.
“I guess 12 or even 13 could do it. Maybe even as much as 15.”
She frowns. She and Schoenfeld go back and forth. She asks him questions. He prods her gently in the right direction. She keeps trying and trying, one approach after another.
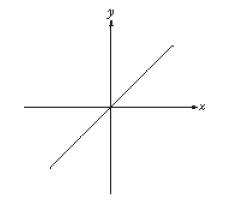
At one point, she types in 20. The line gets a little bit steeper.
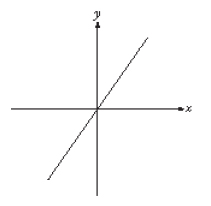
She types in 40. The line gets steeper still.
“I see that there is a relationship there. But as to why, it doesn’t seem to make sense to me....What if I do 80? If 40 gets me halfway, then 80 should get me all the way to the
y
axis. So let’s just see what happens.”
She types in 80. The line is steeper. But it’s still not totally vertical.
“Ohhh. It’s infinity, isn’t it? It’s never going to get there.” Renee is close. But then she reverts to her original misconception.
“So what do I need? 100? Every time you double the number, you get halfway to the
y
axis. But it never gets there...”
She types in 100.