The Amazing Story of Quantum Mechanics (5 page)
Read The Amazing Story of Quantum Mechanics Online
Authors: James Kakalios
It turns out that if you imagine the light striking the metal as analogous to a spray of machine-gun bullets rather than continuous ocean waves, Lenard’s results are completely reasonable. He found that the brighter the light, the more electrons were emitted from the metal. In the machine-gun analogy, brighter light means more bullets per second, which will push more pebbles per second. There will be no time lag between light coming into the metal and electrons coming out, for once a bullet hits a pebble, and it has sufficient energy to knock it up the beach, then of course the effect will be instantaneous. The faster the bullets are traveling, the more energy will be imparted to the pebbles, and the faster they will move up the beach. This corresponds to saying that the greater the energy of each light photon, the more kinetic energy the ejected electrons will have. If the speed of the bullets is too low, they may move the pebbles a little bit but not knock them significantly up the beach. So the threshold effect Lenard observed is also explained. The only snag is that Lenard did not control the energy of his light to vary the energy of the emitted electrons, but rather its frequency. And here we come to the personal catastrophe for Lenard, for the resolution of this last remaining puzzle would cause him distress of a decidedly nonscientific nature. (More on this in a bit.)
If light does indeed consist of discrete packets of energy, what determines the energy of each packet? Planck’s solution to the spectrum of glowing, hot objects was to propose that atoms could lose energy only in finite jumps. In order to get the equations to work out, he assumed that the energy of the jump was proportional to the frequency of the light. That is, the bigger the frequency, the larger the energy of the “quantum step.” Again, I’ll explain why this worked later on. For now the important point is that if the energy is proportional to the frequency, then we can say that the energy is equal to the frequency when multiplied by a constant.
We often deal with situations involving simple proportions, such as the relationship that the longer you drive your automobile at a constant speed, the greater the distance you travel. But you do not measure distance in hours, so to figure out how far you have driven, you need to multiply the hours in the car by a “constant of proportionality,” namely, your constant speed (say 60 miles per hour). Then the product of the time driven (2 hours) and the uniform speed (60 miles per hour) will determine the distance traveled (120 miles). In the same way, the energy of the photon is proportional to the frequency, so that when the frequency is multiplied by a constant of proportionality, it is converted into a quantity of energy. Planck used the letter
h
to represent this constant of proportionality, and everyone who has followed has stuck with that convention, so that
h
is referred to as “Planck’s constant.” The equation for the energy lost by an atom in a glowing object by the emission of light is as follows:
h
to represent this constant of proportionality, and everyone who has followed has stuck with that convention, so that
h
is referred to as “Planck’s constant.” The equation for the energy lost by an atom in a glowing object by the emission of light is as follows:
Energy =
h
× (Frequency)
h
× (Frequency)
Let’s plug some numbers into this simple equation, which is mathematically no different from “distance = (speed) × (time).” One way to measure energy is in a unit termed Joules, named after James Joule, a Scottish physicist who demonstrated the clear equivalence between heat and mechanical work, thereby providing a foundation for the field of thermodynamics. For point of reference, a major-league baseball thrown at 60 miles per hour has a kinetic energy of 53 Joules, while an automobile traveling at 60 miles per hour has a kinetic energy of 600,000 Joules. Frequency is a measure of how many times a periodic function repeats a complete cycle in a given unit of time and is most naturally measured in terms of number of cycles per second. A child’s playground swing that takes 2 seconds to go all the way back and forth will complete only one half of a cycle in a second, so it has a frequency of ½ cycle/second. A much faster playground swing that goes back and forth in 1/10 of a second will complete ten loops in 1 second, and thus has a frequency of 10 cycles/second. Visible light has a frequency of one thousand trillion cycles per second. In order to fit his equation for the spectrum of light given off by a hot, glowing object to the experimentally measured curves, Planck had to set the value of his constant
h
to be
h
= 660 trillionth trillionth trillionth of a Joule-sec, which may seem very small but is in fact very, very, very supersmall.
h
to be
h
= 660 trillionth trillionth trillionth of a Joule-sec, which may seem very small but is in fact very, very, very supersmall.
Planck argued, when justifying his proposed equation, that atoms could lose energy only in finite steps. The closest these energy levels could be was E =
h
× f. For light with a frequency of a thousand trillion cycles per second, this equation gives a spacing between adjacent energy levels of 0.66 millionth trillionths of a Joule. If you compare this energy of a photon to the 50 Joules of kinetic energy of a fastball, you can see that the separation is so small compared to the energy scale of our everyday activities that it’s nearly impossible to observe. Consequently, to take a quantum jump involves a very, very tiny baby step and not some enormous leap. But the size of the step is, in fact, irrelevant, as the important and revolutionary concept introduced by Planck is that there
is
a step.
h
× f. For light with a frequency of a thousand trillion cycles per second, this equation gives a spacing between adjacent energy levels of 0.66 millionth trillionths of a Joule. If you compare this energy of a photon to the 50 Joules of kinetic energy of a fastball, you can see that the separation is so small compared to the energy scale of our everyday activities that it’s nearly impossible to observe. Consequently, to take a quantum jump involves a very, very tiny baby step and not some enormous leap. But the size of the step is, in fact, irrelevant, as the important and revolutionary concept introduced by Planck is that there
is
a step.
As mentioned, Planck was actually somewhat conservative in his quantum hypothesis. For him light was still a continuous electromagnetic wave, like the metaphoric ocean waves washing onto the shore. While he argued that atoms could lose energy only in discrete steps, he was not so bold as to suggest that when they did so the light emitted was also a discrete packet of energy. But as the humorist James Thurber once wrote, “Fools rush in where angels fear to tread. And all the angels are in heaven, but few of the fools are dead.” Perhaps Planck’s hesitancy to extend the quantum graininess to the light itself came from the caution of age. He was a grand old man of forty-two, after all, when he developed his quantum hypothesis. It was left to a younger man of twenty-six to suggest that Lenard’s results could be qualitatively and quantitatively accounted for if light itself were discrete packets, the machine-gun bullets in our beach metaphor, where the energy of each bullet is determined by E =
h
× f. That young man was Albert Einstein.
h
× f. That young man was Albert Einstein.
When Einstein wrote his paper on the “photoelectric” effect, for that is the name used to describe Lenard’s experiment, he was an underemployed twenty-six-year-old patent clerk, third class. That would soon change, for Einstein’s paper was published in 1905, the same year he published his Special Theory of Relativity, followed by a paper describing the equivalence between energy and mass—E = mc
2
—and two other papers on an atomistic explanation for Brownian motion and diffusion processes that would have cemented his reputation as a theoretical physicist of the first order even if he’d done nothing else. Within a few years of the papers’ publication, Einstein would be offered professorships and honors. Most scientists would be thrilled to have their work permanently associated with Einstein, but not Lenard, for one simple reason. Einstein was Jewish, and Lenard was a rabid anti-Semite, to such an extent that Adolf Hitler named Lenard chief of Aryan physics.
2
—and two other papers on an atomistic explanation for Brownian motion and diffusion processes that would have cemented his reputation as a theoretical physicist of the first order even if he’d done nothing else. Within a few years of the papers’ publication, Einstein would be offered professorships and honors. Most scientists would be thrilled to have their work permanently associated with Einstein, but not Lenard, for one simple reason. Einstein was Jewish, and Lenard was a rabid anti-Semite, to such an extent that Adolf Hitler named Lenard chief of Aryan physics.
Thus did his experiments on the influence of ultraviolet light on metals cause a personal catastrophe for Lenard—he spent such effort denouncing Einstein and his interpretation of the photoelectric experiments that his own scientific reputation was ruined, and he is now remembered as much for his bigotry as for his talent as an experimentalist.
Fortunately for Einstein, Lenard was not the only physicist who strongly disagreed with the hypothesis that light was comprised of discrete packets of energy. Nearly all physicists, including the American physicist Robert Millikan, were convinced that light was a continuous wave, and that Einstein’s suggestion (the title of his original paper described his proposal as a “heuristic viewpoint”—which is fancy talk for “not a rigorous solution, but I should get the credit if it turns out to be right”) could not possibly be correct. I say “fortunately for Einstein,” for he did indeed turn out to be right, and it was Millikan who proved it. Millikan was one of the most careful and gifted experimentalists of his day, and he spent ten years trying to show that Einstein was wrong. What he wound up showing, in fact, was that the only possible explanation for the photoelectric effect was Einstein’s hypothesis. Even though he still believed that Einstein’s photon idea was crazy, he stood by his data, which not only unambiguously supported Einstein’s claim but also won Millikan the Nobel Prize. The best advocate for your position is someone who initially doubts but is converted by overwhelming evidence. Einstein’s heuristic viewpoint is now everyone’s viewpoint, and Einstein received the Nobel Prize in Physics, not for his work on relativity or E = mc
2
, but for his theory of Lenard’s photoelectric effect and the introduction of the concept of the light photon (though Gilbert Lewis was the first to use the term “photon,” in 1926).
2
, but for his theory of Lenard’s photoelectric effect and the introduction of the concept of the light photon (though Gilbert Lewis was the first to use the term “photon,” in 1926).
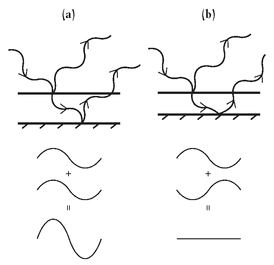
Figure 4:
Sketch of a light wave reflecting from the top and bottom surfaces of an oil slick. In (a) the wavelength and thickness of the slick result in constructive interference of the wave reflected from the top surface, and the wave that travels through the slick reflects from the bottom surface and then exits the slick. For constructive interference the light would be very bright when viewed from the top. In (b) the wavelength and thickness result in destructive interference, in which case no light would be observed from the top surface.
Sketch of a light wave reflecting from the top and bottom surfaces of an oil slick. In (a) the wavelength and thickness of the slick result in constructive interference of the wave reflected from the top surface, and the wave that travels through the slick reflects from the bottom surface and then exits the slick. For constructive interference the light would be very bright when viewed from the top. In (b) the wavelength and thickness result in destructive interference, in which case no light would be observed from the top surface.
Now, for a very long time, a lot of smart people firmly believed that light was a wave, based on a large body of compelling experimental evidence. To cite just one manifestation of the wavelike nature of light, consider the rainbow of colors one sees on an oil slick following a rainstorm. Anyone who lives in a city, or has a messy driveway, may be familiar with the spectrum of light reflected from a thin layer of oil when the ground is wet from a good soaking. In this case the oil is repelled by the water and sits as a thin, freestanding slab that may be only a fraction of a millimeter thick. Rarely will the surface be atomically uniform, so the thickness of the oil slick will vary from location to location. Some of the light striking the oil slick reflects from the top surface, while some penetrates through the oil and then bounces off the oil-water interface. As illustrated by the sketch in Figure 4, if the thickness at one particular spot on the oil slick is exactly equal to one-fourth
9
of the wavelength of one particular color of light, then the light reflected from the top and the light that passed through the slick and bounced back out will be exactly in phase and will add up coherently. The light at that color will thus be particularly bright. Just as two pendulums, if they are swinging side by side with exactly the same frequency and the same phase (so that they both are at the top of the arc or both at the bottom of the swing at the same time), are said to be coherently oscillating, the light that is coherently reflected will add up and give a much more intense color. All other colors will have wavelengths that are not exact multiples of the oil-slick thickness at that location, and they will not add up constructively. This familiar phenomenon is termed “interference” and is a signature property of waves.
9
of the wavelength of one particular color of light, then the light reflected from the top and the light that passed through the slick and bounced back out will be exactly in phase and will add up coherently. The light at that color will thus be particularly bright. Just as two pendulums, if they are swinging side by side with exactly the same frequency and the same phase (so that they both are at the top of the arc or both at the bottom of the swing at the same time), are said to be coherently oscillating, the light that is coherently reflected will add up and give a much more intense color. All other colors will have wavelengths that are not exact multiples of the oil-slick thickness at that location, and they will not add up constructively. This familiar phenomenon is termed “interference” and is a signature property of waves.
We therefore must figure out why light exhibits phenomena that indicate that it is a wave, when it is in fact comprised of photons. It is tempting to argue that the wavelike properties of light are some sort of collective behavior when many photons interact with one another. Oh, if it were only this simple. Consider the following situation: If one sent not a continuous beam of light at the oil slick described above, but single photons, one at a time, then the photons would reflect from the slick, from either the top or bottom surface, and be detected by some sort of light sensor. We would see a single flash at a particular location on our detector when each photon had reflected from the slick. When many such photons had been reflected, the resulting pattern of flashes of light observed would be an interference pattern, identical to what we would see if a continuous beam had been used. That is, even though the photons saw the slick individually, they reflected in such a way that when added together, they yielded a wavelike constructive interference pattern.
Technically, a photon is defined as a quantum of excitation of the radiation field. Well, that certainly clears that up! For our purposes, we will simply accept the notion of photons as discrete entities that move at the speed of light, that have a definite energy (and hence frequency, through E =
h
× f), definite momentum (and hence wavelength, through the relation wavelength = speed of light/ frequency—which we will discuss later on), and definite intrinsic angular momentum (the “spin” of a photon =
h
/2π
,
measured in the direction it is moving). The photon does not spread out as it travels, the way a wave on the ocean’s surface does, but propagates unchanged until it interacts with matter or other photons. There are no simple or satisfying answers to such questions as how big the photon is, or whether it is a wave or a particle. If you find this confusing—join the club! It’s a big club with some rather distinguished members. The president of this club would be the scientist who introduced the photon concept in the early days of quantum mechanics. As Albert Einstein reflected, “All the fifty years of conscious brooding have brought me no closer to the answer to the question: What are light quanta? Of course today every rascal thinks he knows the answer, but he is deluding himself.”
h
× f), definite momentum (and hence wavelength, through the relation wavelength = speed of light/ frequency—which we will discuss later on), and definite intrinsic angular momentum (the “spin” of a photon =
h
/2π
,
measured in the direction it is moving). The photon does not spread out as it travels, the way a wave on the ocean’s surface does, but propagates unchanged until it interacts with matter or other photons. There are no simple or satisfying answers to such questions as how big the photon is, or whether it is a wave or a particle. If you find this confusing—join the club! It’s a big club with some rather distinguished members. The president of this club would be the scientist who introduced the photon concept in the early days of quantum mechanics. As Albert Einstein reflected, “All the fifty years of conscious brooding have brought me no closer to the answer to the question: What are light quanta? Of course today every rascal thinks he knows the answer, but he is deluding himself.”
Other books
Bleeder by Smoak, Shelby
The Willows at Christmas by William Horwood
Shadow of a Spout by Amanda Cooper
Secrets of a Summer Night (Stone Gap Mountain) by Stockham, Kay
Fire And Ash by Nia Davenport
A Knight’s Enchantment by Townsend, Lindsay
The Black Palmetto by Paul Carr
Teen Idol by Meg Cabot
Ghostwriter by Travis Thrasher
If I Die by Rachel Vincent