The Amazing Story of Quantum Mechanics (22 page)
Read The Amazing Story of Quantum Mechanics Online
Authors: James Kakalios
Up to now, we have made extensive use of the first two quantum principles listed in Section 1: that light consists of discrete packets of energy termed photons, and that there is a wave associated with the motion of all matter. We have not needed to invoke the third principle: that all matter and light has an internal rotation that corresponds to discrete values of angular momentum. We would have needed this principle in order to understand details of how the electronic energy states in an atom are arranged, but for our purposes there was no call to head into this set of weeds. However, we cannot avoid a certain amount of weediness now, not if we wish to understand the basis for the semiconductor age and the foundations of the upcoming nanotechnology revolution.
In Chapter 4 we discussed the intrinsic angular momentum that all subatomic particles possess, termed spin. Associated with this internal spin is a magnetic field, so that every electron, proton, and neutron can also be considered a tiny bar magnet, with a north and a south pole (Figure 10b). While the concept of spin was introduced to account for experimental observations indicating that electrons possessed a built-in magnetic field, one cannot ascribe this magnetic field to a literal, physical rotation of the subatomic particles as if they were ballerinas. It is indeed confusing to imagine an intrinsic angular momentum, as integral to the properties of the electron and as real as its charge or mass, that is not associated with a literal rotation. Nevertheless, spin is the term that has stuck, and we adhere to this nomenclature, as we are nothing if not slaves to convention.
As mentioned in Chapter 4, the intrinsic angular momentum of electrons is exactly
ħ
/2 (recall that
ħ
is defined as
h
/2π).
46
The “spinning” electron can have an intrinsic angular momentum of either +
ħ
/2 or -
ħ
/2, just as a real spinning ballerina can twirl either clockwise or counterclockwise. No other intrinsic angular momentum values are possible for electrons (or protons or neutrons). The collective behavior of quantum particles that have a spin of ±
ħ
/2 was first worked out by Enrico Fermi and Paul Dirac in the 1920s. In honor of their contribution, physicists refer to all spin
ħ
/2 particles as obeying “Fermi-Dirac statistics,” or by the shorter nickname of fermions. (Fermi got the sweet part of this deal—the fact that electrons are spin
ħ
/2 particles, and thus are fermions, has led to a host of quantities in solid-state physics as being labeled with his name—Fermi Energies, Fermi Surfaces, and so on—even though he made few direct contributions to this field of physics.)
ħ
/2 (recall that
ħ
is defined as
h
/2π).
46
The “spinning” electron can have an intrinsic angular momentum of either +
ħ
/2 or -
ħ
/2, just as a real spinning ballerina can twirl either clockwise or counterclockwise. No other intrinsic angular momentum values are possible for electrons (or protons or neutrons). The collective behavior of quantum particles that have a spin of ±
ħ
/2 was first worked out by Enrico Fermi and Paul Dirac in the 1920s. In honor of their contribution, physicists refer to all spin
ħ
/2 particles as obeying “Fermi-Dirac statistics,” or by the shorter nickname of fermions. (Fermi got the sweet part of this deal—the fact that electrons are spin
ħ
/2 particles, and thus are fermions, has led to a host of quantities in solid-state physics as being labeled with his name—Fermi Energies, Fermi Surfaces, and so on—even though he made few direct contributions to this field of physics.)
Consider two fermions, such as electrons. It really is true that all electrons look alike. This is not the prejudiced opinion of an anti-Fermite, but a reflection of the fact that all fundamental particles of a given type are identical. There is no way to distinguish or differentiate between electrons, for example. Similarly, all protons are identical, as are all neutrons. These three subatomic particles have different masses and electrical charges, so they can be distinguished from one another. But if we bring two electrons so close to each other that their de Broglie waves overlap, then no observable property can possibly depend on which electron is which.
If I toss a rock into a pond, a series of concentric circular ripples forms (Figure 29a). When I toss two rocks into the water a small distance apart, each forms its own set of ripples, and the combined effect is a complicated interference pattern (Figure 29b). At some points the ripples from each rock add up coherently and create a larger disturbance on the water’s surface than generated by each rock separately. At other locations the two ripples are exactly out of phase, so that one ripple is at a peak while the other stone’s wave is at a trough, and the two exactly cancel each other out. Taken together, the resulting pattern is more than just a doubling of the result of one stone’s concentric ripples.
All objects have a quantum mechanical wave function. When two electrons are brought together such that their wave functions intersect, then they are described by a two-electron wave function. In the case of two rocks tossed into the pond, if the stones are identical and both are tossed into the water in the exact same way, then the interference pattern that is observed does not depend on which rock was tossed on the left and which on the right. Similarly, in atomic physics, nothing that we can measure, such as the wavelength of light emitted from transitions between quantized energy states, can depend on any artificial labeling of the electrons. In the case of the stones in the water, they are indeed distinguishable, for we can refer to the stone on the left and the stone on the right in a meaningful way. Heisenberg tells us that it is fruitless to try to specify the location of the electron more precisely than the extent of its de Broglie wave. When two de Broglie waves overlap, concepts such as “left” and “right” become irrelevant, and all we have is the composite two-electron wave function.

Figure 29:
Cartoon of the wave patterns observed on the surface of a pond when one rock is tossed into the water (a), and when two rocks are simultaneously tossed, near but not touching each other (b).
Cartoon of the wave patterns observed on the surface of a pond when one rock is tossed into the water (a), and when two rocks are simultaneously tossed, near but not touching each other (b).
Say I have two electrons, which I will creatively call electron 1 and electron 2. I bring them together so that their wave functions intersect. The electrons are indistinguishable, and no measurements can depend on which one is labeled “electron 1” and which one is “electron 2.” Are there any differences at all between them at this point? Indeed yes! The two electrons, 1 and 2, have identical electrical charges and identical masses, but they can have different intrinsic angular momentum. Both electron 1 and electron 2 can have spin values of +
ħ
/2, or both could have a spin value of -
ħ
/2, or one could have a spin of +
ħ
/2 while the other has spin of -
ħ
/2. These different values of spin will be crucial for understanding solid-state physics.
ħ
/2, or both could have a spin value of -
ħ
/2, or one could have a spin of +
ħ
/2 while the other has spin of -
ħ
/2. These different values of spin will be crucial for understanding solid-state physics.
Think about a ribbon, one side of which is black and the other of which is white. The ribbon represents a single electron, and if I hold the ribbon so that the white side is facing you, it indicates that the electron’s spin is +
ħ
/2, while if the black side is shown this means the spin is -
ħ
/2. Now, if I hold two ribbons far away from each other, I can easily distinguish them—one is on the right and the other is on the left. Bring them so close that their waves overlap and I can no longer tell them apart. In this case I can describe them both with a single, longer ribbon. I can still represent the case where one electron has spin of +
ħ
/2 and the other has spin of -
ħ
/2, by having my right hand hold the ribbon with the white side facing out and my left hand hold the ribbon’s black side facing out. Figure 30 shows a ribbon where both ends have the white side facing out, indicating that both electrons have a spin of +
ħ
/2. The arguments presented in this figure are a modification of those made by David Finkelstein, as described in Richard Feynman’s essay “The Reason for Antiparticles.” I need hardly stress that the “ribbon” is simply a metaphor that will, I hope, assist in the visualization of a two-particle wave function, and is not intended as a literal representation.
ħ
/2, while if the black side is shown this means the spin is -
ħ
/2. Now, if I hold two ribbons far away from each other, I can easily distinguish them—one is on the right and the other is on the left. Bring them so close that their waves overlap and I can no longer tell them apart. In this case I can describe them both with a single, longer ribbon. I can still represent the case where one electron has spin of +
ħ
/2 and the other has spin of -
ħ
/2, by having my right hand hold the ribbon with the white side facing out and my left hand hold the ribbon’s black side facing out. Figure 30 shows a ribbon where both ends have the white side facing out, indicating that both electrons have a spin of +
ħ
/2. The arguments presented in this figure are a modification of those made by David Finkelstein, as described in Richard Feynman’s essay “The Reason for Antiparticles.” I need hardly stress that the “ribbon” is simply a metaphor that will, I hope, assist in the visualization of a two-particle wave function, and is not intended as a literal representation.
The ribbon in Figure 30 represents a two-electron wavefunction with both electrons having a spin of +
ħ
/2. The fact that the two electrons are so close that they are described by a single wave function is represented by the fact that I use one ribbon for both electrons. Any change to one electron is thus communicated to the other. What if I switch their positions, so that I move the right-hand side to the left and the left passes to the right? If I do this—without letting go of either end of the ribbon—then by switching their locations, I will add a half twist to the ribbon (Figure 30b). This is
not
the same situation I started with—as the ribbon has a half twist that it did not have before switching their positions. One can tell from inspection of the ribbon that a swap from left to right has occurred.
ħ
/2. The fact that the two electrons are so close that they are described by a single wave function is represented by the fact that I use one ribbon for both electrons. Any change to one electron is thus communicated to the other. What if I switch their positions, so that I move the right-hand side to the left and the left passes to the right? If I do this—without letting go of either end of the ribbon—then by switching their locations, I will add a half twist to the ribbon (Figure 30b). This is
not
the same situation I started with—as the ribbon has a half twist that it did not have before switching their positions. One can tell from inspection of the ribbon that a swap from left to right has occurred.
And that’s it. That’s the heart of Fermi-Dirac statistics, which governs the way electrons interact with one another and is the basis of the periodic table of the elements, chemistry, and solid-state physics.
How do I mathematically combine the wave functions for two electrons so that switching their order changes the situation, but making another swap restores the original state? Easy: Let the two-electron wave function Ψ be described as the difference of two functions, A and B, that is, Ψ = A - B, where A and B each depend on the one-electron wave functions at positions 1 and 2.
47
As in switching the two ends of our metaphoric ribbon, let’s move the electron that was at one position to the location of the other electron, and conversely. In this case the wave function would be written as Ψ = B - A. The process of switching the positions of the two electrons is the same as multiplying the original two-electron wave function by (-1). If I want to get back to the original configuration, I do another switch, which brings me to Ψ = A-Bagain.
47
As in switching the two ends of our metaphoric ribbon, let’s move the electron that was at one position to the location of the other electron, and conversely. In this case the wave function would be written as Ψ = B - A. The process of switching the positions of the two electrons is the same as multiplying the original two-electron wave function by (-1). If I want to get back to the original configuration, I do another switch, which brings me to Ψ = A-Bagain.
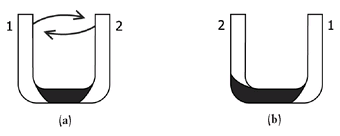
Figure 30:
Cartoon sketch of a ribbon with different colors on each side, where the ribbon is presented so that each end displays the same side (the white side in this case). Switching the two ends results in a half-twist in the ribbon. Only another rotation creates a full twist in the ribbon that can now be removed by flipping one side of the ribbon twice.
Cartoon sketch of a ribbon with different colors on each side, where the ribbon is presented so that each end displays the same side (the white side in this case). Switching the two ends results in a half-twist in the ribbon. Only another rotation creates a full twist in the ribbon that can now be removed by flipping one side of the ribbon twice.
Nothing that I can measure should depend on which electron I label at position 1 and which one is at position 2. Now, there’s no problem with having a two-electron wave function written as A - B. The fact that switching the positions is the same as multiplying the wave function by -1 will not affect any measurement we make. Remember that while the wave function Ψ contains all the information about the quantum mechanical system, it is the wave function
squared
Ψ
2
that gives us the probability of finding the object at some point in space and time.
48
It is also the wave function squared Ψ
2
that is used in calculating the average position (we add up all possible positions when multiplied by the probability—Ψ
2
—of the electron being at that position), the average momentum, and so on. And since the square of negative one is (-1)
2
= (-1) × (-1) = +1, then Ψ = A-Bis a physically valid way to represent the two spin
ħ
/2 electrons. In Chapter 8, Dr. Manhattan’s ability to change his size at will was ascribed to the fact that the Schrödinger equation is linear. This really becomes important here. Only for a linear equation will it be true that if A or B are separately solutions, then Ψ = A-B(or Ψ = A + B, discussed in the next chapter), will also be a valid solution of the Schrödinger equation.
squared
Ψ
2
that gives us the probability of finding the object at some point in space and time.
48
It is also the wave function squared Ψ
2
that is used in calculating the average position (we add up all possible positions when multiplied by the probability—Ψ
2
—of the electron being at that position), the average momentum, and so on. And since the square of negative one is (-1)
2
= (-1) × (-1) = +1, then Ψ = A-Bis a physically valid way to represent the two spin
ħ
/2 electrons. In Chapter 8, Dr. Manhattan’s ability to change his size at will was ascribed to the fact that the Schrödinger equation is linear. This really becomes important here. Only for a linear equation will it be true that if A or B are separately solutions, then Ψ = A-B(or Ψ = A + B, discussed in the next chapter), will also be a valid solution of the Schrödinger equation.
Right off the bat there’s a big consequence of writing the two-electron wave function as Ψ = A - B. What happens if I try to make both electrons be at the same location, or have both electrons in the same quantum state (when they are close enough to overlap and are described by a single two-electron wave function), so that the function A is equal to the function B? Then the two-electron wave function would be Ψ = A-B= 0 when A = B. If Ψ = 0, then the square of the wave functionΨxΨ= Ψ
2
= 0 as well. Physically, this means that the probability of finding two electrons at the same place in the exact same quantum state is zero—as in, this will
never
happen. Recall in Chapter 8 our discussion of quantum mechanical tunneling. In a tunneling situation an electron in one metal, separated by the vacuum of empty space from another metal and not having sufficient energy to arc or jump from one metal to another, may nonetheless find itself in the second material. We pointed out that even though the probability for the electron to be outside of metal may be very small, as in one chance out of a trillion, there was still
some
chance of finding the electron in the second metal. The only time something will
never
be observed is if the probability of it happening is exactly zero. If something can never be observed, in physics we say that it is forbidden.
2
= 0 as well. Physically, this means that the probability of finding two electrons at the same place in the exact same quantum state is zero—as in, this will
never
happen. Recall in Chapter 8 our discussion of quantum mechanical tunneling. In a tunneling situation an electron in one metal, separated by the vacuum of empty space from another metal and not having sufficient energy to arc or jump from one metal to another, may nonetheless find itself in the second material. We pointed out that even though the probability for the electron to be outside of metal may be very small, as in one chance out of a trillion, there was still
some
chance of finding the electron in the second metal. The only time something will
never
be observed is if the probability of it happening is exactly zero. If something can never be observed, in physics we say that it is forbidden.
Other books
All In: Double or Nothing by Lane Hart
The Heir Agreement by Leon, Kenzie
The Wishing Tide by Barbara Davis
Forever and Always by E. L. Todd
Punishment with Kisses by Diane Anderson-Minshall
Lady Killer by Michele Jaffe
Forbidden by Lauren Smith
Lightning by Danielle Steel
Time Traders by Andre Norton
Grace by Elizabeth Nunez